
In 1687 Isaac Newton published the Philosophiæ Naturalis Principia Mathematica that described both the laws of motion and the law of universal gravitation. The Principa thus laid the foundation of classical mechanics. Newtons theory was unchallenged until Albert Einstein published his theories of relativity a series of papers between 1905 and 1916. According to the Special and the General theory of relativity Newtons laws are only approximate and receive corrections when objects move at large velocity or are in the vicinity of strong gravitational fields.
In 1922, soon after the publication of the theory of General Relativity, Alexander Friedmann showed that viable models of the Universe were expanding in time. This was observationally confirmed by Edwin Hubble in 1929, and this marked the beginning of physical cosmology.
It may then come as a surprise that the theoretical predictions for the large scale structure that we observe in the Universe today are computed using Newtons laws and not Einsteins relativistic theory. Going beyond this Newtonian approximation, even in the presence of massive neutrinos, is precisely the objective of my research proposal.
Project title:
Neutrino simulations under the spell of General Relativity
Area of research:
Cosmology
Fellowship period:
1 Oct 2017 – 30 Sep 2019
Fellowship type:
AIAS-COFUND Marie Skłodowska-Curie fellow

This fellowship has received funding from the European Union’s Seventh Framework Programme for research, technological development and demonstration under the Marie Skłodowska-Curie grant agreement No 609033 and The Aarhus University Research Foundation.

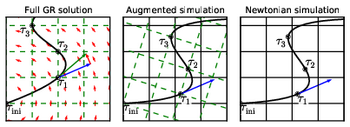
Figure 1: Illustration of an augmented Newtonian simulation. The non-Newtonian force (red arrows) impacts the dark matter trajectories. For illustration purposes the e↵ect is a time-independent rotation, but in practice it will be more complicated. The velocity contribution from Newtonian forces shown in blue dominate. The left plot shows the full solution in GR while the right plot shows the trajectory solved in an unmodified Newtonian simulation. The middle plot shows how the non-Newtonian part can be absorbed into the coordinates.